Seems the infamous mathematical probability distribution function, the Gaussian Copula, is at the forefront of controversy once again. It seems those financial engineers, the Quants, the ones who use advanced probability and statistics to model financial markets, upon whose work many derivatives are based, knew the use of Gaussian Copulas was fundamentally flawed.

The quant who told us that the Gaussian copula was ‘not a model’ went on to explain what he meant: ‘it doesn’t satisfy the law of one price [in other words, the absence of arbitrage opportunities]. It … can give you inconsistencies and arbitrages very easily. You’re not computing values … as expectations under some well-defined measure.’

It is important to note that the quant who said this had made important technical contributions to the development of the Gaussian copula family of models. Others were even more critical. ‘Copulas are generally an early doodling activity in an area’, said another quant in a January 2009 interview. ‘They are a simple trick to allow yourself to preserve the marginals [default probabilities for individual corporations] and to induce some sort of coupling.’ Copulas ‘are perceived as a hack’, he said, despite having ‘superficially attractive properties like being able to perfectly reproduce markets’.

We covered the properties of Gaussian Copulas previously, pointing to their mathematical properties being invalid to price a CDO. In particular, the properties of CDSes, credit default swaps as inputs were not valid for they are not a 1:1 relationship, i.e. one price as stated above. Folks, in a nutshell, one cannot allow just anyone to buy credit default swaps, which are correlated to an underlying asset, and not risk creating an economic maelstrom. Credit default swaps do not require buyers to have a stake in the underlying assets they insure. This is a disconnect and turns the 1:1 relationship, i.e. a normal distribution property, on it's head. It's right there in the mathematical model's assumptions and properties, yet unfortunately advanced probability and statistics are not everyone's daily cup of tea. Few seem to realize the flaws are in the mathematical properties and their misuse and misapplication.

Yet it seems now Copulas are being forgiven anyway. The latest misunderstanding is believing Gaussian Copulas were not used in ABS CDOs at all. This isn't true, they were, just those gamma inputs into the probability distribution functions were changed. The paper, The Formula That Killed Wall Street’? The Gaussian Copula and the Material Cultures of Modeling is what's causing the ruckus. Here is the excerpt blaming the credit rating agencies instead of the Copula model:

The critical path by which the Gaussian copula was implicated in the credit crisis was via rating agencies, in particular in the rating not of CDOs based on pools of corporate debt `but of ‘ABS CDOs’. These are CDOs in which the underlying assets are asset-backed securities (ABSs), specifically mortgage-backed securities. These were introduced somewhat later than corporate-debt CDOs, and originally were a small-scale business: of the 283 CDOs issued in 1997-1999, only eight were ABS CDOs (Newman et al., 2008: 34, exhibit 1). By the time ABS CDOs started to become large-scale (from 2001 onwards), the rating agencies already had in place an organizational division of labour. Both CDOs and ABSs fell within the remit of their structured finance departments, but those departments had separate groups rating CDOs, on the one hand, and ABSs on the other (author ref.). The new ABS CDOs were therefore evaluated by the rating agencies in two temporally and organizationally separate steps. First, the underlying mortgage-backed securities or other ABSs were rated by the groups handling ABSs, and then the overall CDO structure was rated by the CDO groups. Instead of considering ABS CDOs as radically different instruments that required an altogether new form of evaluation, the CDO groups simply made relatively modest modifications to their existing techniques for analyzing CDOs with pools consisting of corporate debt. From late 2001 onwards, those techniques increasingly involved the use of models in the Gaussian copula family, albeit – as noted above – usually one-period models analogous to CreditMetrics, not fully-fledged copulas of the kind introduced by Li. With little econometric data to draw upon (empirically estimating the correlation between ABSs is an even harder econometric problem that estimating corporate correlations), the CDO groups employed largely judgment-based ABS correlation estimates broadly similar to those they used for the analysis of corporate CDOs. When, for example, Standard & Poor’s introduced its new one-period Gaussian copula system, CDO Evaluator, in November 2001 the same correlation (0.3) was used for the correlation between ABSs from the same sector (for example, ABSs based on subprime mortgages) as was used for the correlation between corporations in the same industry (Bergman 2001).

Folks, let's be clear, it is the misuse of a Copula based model that is at fault here and regardless of trading desks relying on credit rating agencies, it was the incorrect application of a model, based on a Gaussian Copula that is at the structural heart of the ABS CDO disaster. The fact anybody, anybody at all used credit default swaps as inputs into this model is where they broke it. That said, the other uses of Gaussian Copulas are gonna also have problems. As been said many times, the world is not a bell curve, one cannot assume because 80% of the time events will happen, to base a model that the odd occurrence will not ever happen is wrong. Not designing safeguards for that one in a million chance is a mistake and that's where things go wrong.

Felix Simon stands by his claim that models based on the Copula function wreak havoc around the globe:

But the fact is that the Gaussian copula function was invidious, and did cause enormous losses all over the world. The way I like to think about it is that value-at-risk allowed banks to ignore tail risk, and the Gaussian copula function did a magnificent job in maximizing that tail risk. The two together were lethal. And while there are surely many other models which inhabit Wall Street in much the same way, I sincerely hope that none of them are remotely as dangerous as the Gaussian copula function turned out to be.

Math Babe points to some fairly suspect pecking order hierarchy in the world of Wall Street that could cause flawed models, based on improperly applied probability distribution functions, to become widely used, the warnings from quantitative analysts unheeded. Quants are at the bottom of the corporate pecking order. Trades, tied to derivatives models, give big fat bonuses. These mathematicians' results are probably not understood and there is no corporate power structure, checks and balances, to even heed Quants dire warnings on incorrect use of probability models.

How many places employing quants to create risk or hedging models have their quants actually in charge of stuff? Very, very few is the answer. The quants are not in charge, they rarely have real power, and as soon as they produce something semi-functional and useful, they no longer own that thing – it’s been taken away from them and is owned by the real power brokers.

When greed meets math some ground rules need to be set. One might look at quantitative analysis, mathematical modeling, financial engineering as analogous to building a large bridge. Both use mathematics, theory, one to make profits and model risk, the other to transport large numbers of machines and people over dramatic heights, usually spanning large bodies of water.

Engineering has national and international standards bodies. Engineering has testing. Engineering has safety requirements. Many of these safety standards, procedures, testings and standards bodies came about after engineering disasters. Take the case of the Columbia disaster, we had investigations and joint Congressional hearings. Can we say that about derivatives mathematics, financial models?  Seems not!

There appears to be only one hearing on derivatives models, the House Science Committee hearing, The Science of Insolvency. Yet, Copulas are only mentioned in passing, as if they are just too big of a word.

Any engineer worth their salt knows it is a rare day when one can publish an Academic paper and takes those results directly into production. There are processes, testing, standardization & proofing.

Even the Society of Quantitative Analysis does not mention true standards bodies or affiliated with government sponsored standards bodies. There are liabilities, lawsuits and regulatory agencies, yet seemingly no standards body for the derivatives model itself. Derivatives are financial engineering and it seems the safety, validation and standards bodies normative to any other engineering discipline are currently not being applied.

It is one thing for a bunch of journalists and bloggers to write about a complex multivariate probability model, with half of 'em getting the mathematical characterizations incorrect, while earning an A for effort. It's quite another for a profession to start policing itself. Here is where the Qaunts are coming up short, their actual financial engineering process. Hey, if something is a flawed model, one does not whisper under one's breath that it's flawed. They need to stand up and plain remove that model from production, from use. Quants need a derivatives recall! Take it off of the market. Don't think one can reform the OTC derivatives market without addressing flawed models upon which those derivative are based.

To wit, we have a paper from the Bank of International Settlements basically saying financial institutions, banks are at it again. Yes, more risky derivatives as we pointed out when Moody's downgraded 15 Banks. The BIS also reports banks believe they will be bailed out by taxpayers if their house of cards, risky derivatives trades crash and burn.

Banks and prudential authorities still face tough challenges in securing financial stability. Banks need to further strengthen capital and liquidity positions to regain markets' confidence. To expedite this process, authorities should ensure that institutions recapitalise and recognise losses on problematic investments. Authorities everywhere must complete their consistent and timely implementation of the agreed Basel III standards and ensure that robust regulation extends to currently unregulated intermediaries. Meanwhile, regulators in rapidly growing economies should be aware of the potentially destabilising risk-taking encouraged by buoyant local markets. The long-term objective of policy must be to pave the way to a robust business model of banking featuring strong and transparent balance sheets, self-sustaining international operations, and stable profits that do not rely on official support.

It's fairly unbelievable this is never talked about. We wouldn't let people drive over a flawed bridge design, so why do we allow trillions of dollars to be at risk flowing through faulty derivatives models?

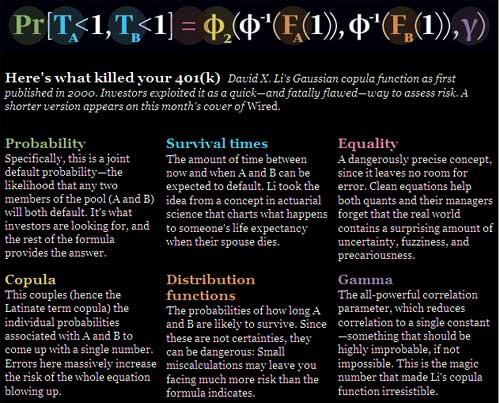
Below is the very good original layman's explanation of a Gaussian Copula, from the Wired Magazine article.

Thanks to Naked Capitalism for alerting us to the returning Copula controversy.